
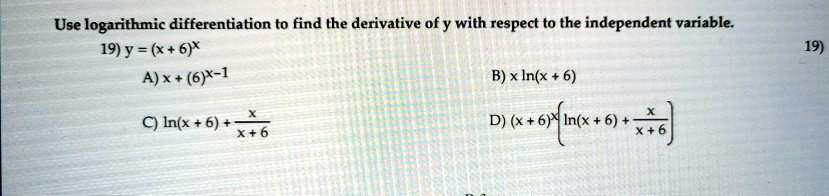
log 10 x is frequently denoted logx, and is called the common logarithm.' Properties of Logarithms: 1. log e x is frequently denoted lnx, and called the natural logarithm.' (e 2:71828). For example, log 2 8 3 since 23 8 and log 3 1 3 1 since 3 1 3. Notice how we haven"t said what the base is. For a constant a with a > 0 and a 6 1, recall that for x > 0, y log a x if ay x. Logarithms can be used to help solve equations of the form a x = b by "taking logs of both sides". If we are given equations involving exponentials or the natural logarithm, remember that you can take the exponential of both sides of the equation to get rid of the logarithm or take the natural logarithm of both sides to get rid of the exponential. It therefore follows that the integral of 1/x is ln x + c. Ln x is also known as the natural logarithm. Most calculators can only work out ln x and log 10x (usually just written as "log" on the button) so this formula can be very useful. This is a very useful way of changing the base (in this formula, the base does matter!). This is because for the laws of logarithms, it doesn"t matter what the base is, as long as all of the logs are to the same base.Īnother important law of logs is as follows. NB: In the above example, I have not written what base each of the logarithms is to. The properties of indices can be used to show that the following rules for logarithms hold: Remember that e is the exponential function, equal to 2.71828… It is generally recognised that this is shorthand: You may often see ln x and log x written, with no base indicated.

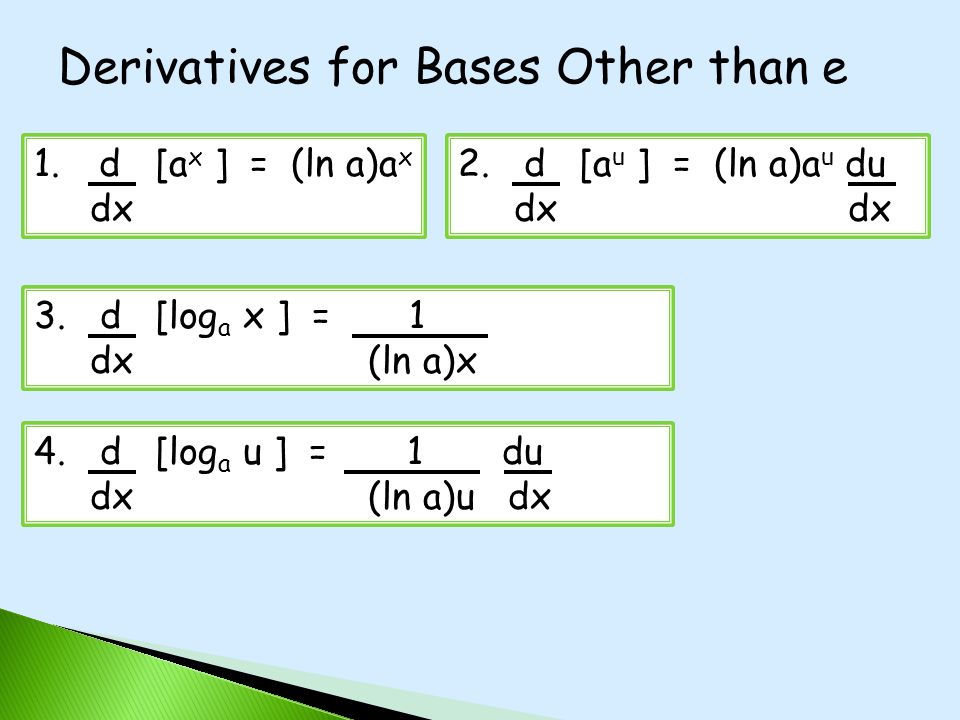
Logarithms are another way of writing indices. Notice that lnx and e x are reflections of one another in the line y = x. In the diagram, e x is the red line, lnx the green line and y = x is the yellow line. Like most functions you are likely to come across, the exponential has an inverse function, which is log ex, often written ln x (pronounced 'log x'). In other words:īecause of this special property, the exponential function is very important in mathematics and crops up frequently. The exponential function, written exp(x) or e x, is the function whose derivative is equal to its equation. It is read as the derivative of a raised to the power of x with respect to x is equal to the product of a x and ln. common logs, log10, are typically logarithms base 10. The differentiation of exponential function with respect to a variable is equal to the product of exponential function and natural logarithm of base of exponential function. For any, the elements of which affect are those which do not lie on row or column. A function fis said to be even if and only if, f(x)f(x) for all x belonging to A. This means that the first term above reduces to. On the other hand, by the cofactor expansion of the determinant,, so by the product rule, These terms are useful because they related to both matrix determinants and inverses. The adjugate matrix of, denoted, is simply the transpose of.The cofactor matrix of, denoted, is an matrix such that.the minor of, denoted, is the determinant of the matrix that remains after removing the th row and th column from.(An alternate proof is given in Section A.4.1 of Steven Boyd’s Convex Optimization.)īefore we get there, we need to define some other terms. (Here, we restrict the domain of the function to with positive determinant.) The most straightforward proof I know of this is direct computation: showing that the th entry on the LHS is equal to that on the RHS. įor some functions, the derivative has a nice form. When the logarithmic function is given by: f ( x) log b ( x) The derivative of the logarithmic function is given by: f ' ( x) 1 / ( x ln ( b) ) x is the function argument. For a function, define its derivative as an matrix where the entry in row and column is.


 0 kommentar(er)
0 kommentar(er)
